Speaker
Description
Modern developments in nanotechnology allow production of artificial curvilinear nanostructures [1] as well as modification of natural nanosized objects with curved geometry [2]. Such objects attract considerable interest for both theoretical and experimental investigation due to their outstanding physical properties. Also, in the last few years interest in antiferromagnets (AFM) has increased due to their advantages over ferromagnets, namely higher characteristic frequencies and absence of macroscopic magnetization.
However, there is still a need for fundamental investigation of AFM curvilinear systems.
Our work focuses on the investigation of equilibrium states and linear dynamics of AFM textures in curvilinear AFM one-dimensional spin chains. We show, that the magnetic dipole-dipole interaction results in a hard-tangential anisotropy in a spin chain. In our study we consider two sublattice collinear AFM with slowly varying AFM textures in the frame of generalized sigma-model approach.
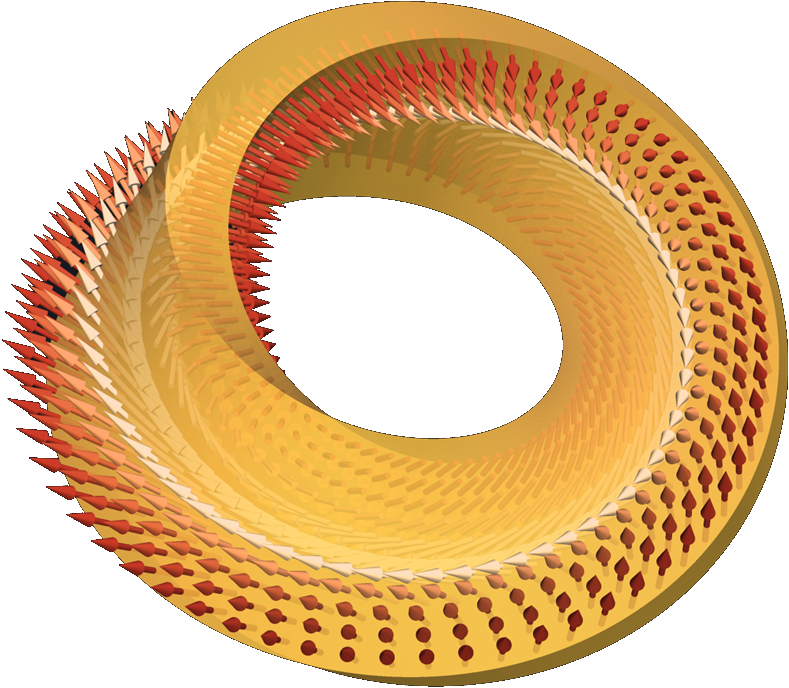
We apply the approach [3, 4], recently developed for ferromagnets, to describe equilibrium AFM textures in helix-shaped and ring-shaped spin chains. The binormal distribution of the Neel vector field corresponds to the ground state of an AFM ring-shaped spin chain whereas AFM helix-shaped spin chain has two equilibrium states: (i) quasi-homogeneous and (ii) quasi-binormal. The state (i) appears for helices with curvature less than torsion and state (ii) vice versa. The software package used to determine stability regions of both ground states was SLaSi simulator [5].
We also describe linear excitations of the equilibrium states in helix-shaped and ring-shaped spin chains. We show that magnon spectrum in the AFM ring-shaped spin chain is discrete and each frequency state is four times degenerated. Magnon spectrum of helix-shaped AFM spin chain is characterized by four dispersion branches.
[1] R. Streubel, P. Fischer, F. Kronast, V. P. Kravchuk, D. D. Sheka, Y. Gaididei, O. G. Schmidt and D. Makarov, J. Phys. D, 49, 363001, (2016).
[2] K. Mizoguchi, S. Tanaka, M. Ojima, S. Sano, M. Nagatori, H. Sakamoto,
Y. Yonezawa, Y. Aoki, H. Sato, K. Furukawa, T. Nakamura, Journal of the Physical Society of Japan, 76, 043801, (2007).
[3] Y. Gaididei, V. P. Kravchuk, D. D. Sheka, Phys. Rev. Lett., 112, 257203, (2014); D. D. Sheka, V. P. Kravchuk, Y. Gaididei, J. Phys. A, 48, 125202, (2015).
[4] D. D. Sheka, V. P. Kravchuk, K. V. Yershov and Y. Gaididei, Phys. Rev. B, 92, 054417, (2015); O. V. Pylypovskyi, V. P. Kravchuk, D. D. Sheka, D. Makarov, O. G. Schmidt and Y. Gaididei, Phys. Rev. Lett., 114, 197204, (2015); V. P. Kravchuk, U. K. Rößler, O. M. Volkov, D. D. Sheka, J. van den Brink, D. Makarov, H. Fuchs, H. Fangohr and Y. Gaididei, Phys. Rev. B, 94, 144402, (2016).
[5] O. V. Pylypovskyi, D. D. Sheka, V. P. Kravchuk, Y. Gaididei, Journal of Magnetism and Magnetic Materials, 361, 201 – 205, (2014).